“I believe that we do not know anything for certain, but everything probably.”
—Christiaan Huygens

Have you ever wondered how health insurance premiums are calculated or why healthcare is so expensive? Or what led to the financial crisis of 2008? Or whether nuclear power is safe? The answers to these questions require an understanding of probability, which is the best tool that we have for coping with an uncertain world. In fact, an understanding of probability is required for professionals in a large number of fields, including data science, finance, engineering, biology, chemistry, medicine and actuarial science.

I am glad to announce the launch of Introduction to Probability, a free interactive course aiming to help you learn probability intuitively, from simple to advanced concepts. Anyone who wants to learn probability for the first time, needs a refresher or is looking to apply probability professionally will find great value in this course. It will help students understand and use randomness and random variables.

Clicking the following will take you directly to the course, where you can immediately venture into the uncertain world of probability.

Motivation from History

Probability can be traced all the way back to the first game of chance, but these were only first studied in the 1560s by the mathematician Gerolamo Cardano. Even in its infancy, probability was fundamentally applied: Cardano used this knowledge to earn more when gambling. It’s only a century later, in 1654, that probability theory was made public through a correspondence between Pierre de Fermat and Blaise Pascal about a gambling question known today as the problem of points.

Despite its questionable and amusing origins in games of chance, probability is nowadays crucial in many cutting-edge domains such as artificial intelligence, data science, quantum physics and stock exchanges. Whether you aim to revolutionize our world or stack the odds in your favor in a game of poker, probability will help and inform you to make better decisions.

Overview

The course begins with an introduction to basic probability definitions and prerequisite knowledge. Students will then intuitively learn to construct and manipulate any random variable, the central concept of this course. This is followed by a lesson for each important random variable and its applications, as well as their advanced generalizations. The lessons conclude with important theorems and approximations explaining theoretically previously seen notions.

Here is a bit of a sneak peak of the lesson contents:

This course has 25 lessons. The order of lessons is a suggestion that aims to build intuition; however, lessons can be studied independently. You can finish watching all of the videos and completing the six short quizzes in four hours, but I recommend attempting all exercises and reading their solutions to cement your knowledge, which may take you an additional three hours.

While concepts from Introduction to Calculus and Introduction to Linear Algebra are mentioned, these are not necessary, and anyone with minimal knowledge of Wolfram Language and high-school-level math can excel in this course.

Lessons

This course is built around a collection of 25 lessons that aims to build the student’s problem-recognition and problem-solving abilities. Indeed, probability problems are everywhere, and the hardest part is often recognizing the type of problem in order to use the right solution.

In the creation of the course, special care was taken to pronounce place names as they would be in their original languages. Moreover, examples and exercises are geared toward fields where probability is frequently used professionally, like data and actuarial science.

This course is aimed more at practitioners of probability than mathematics students; therefore, a greater emphasis is given to applications rather than proofs.

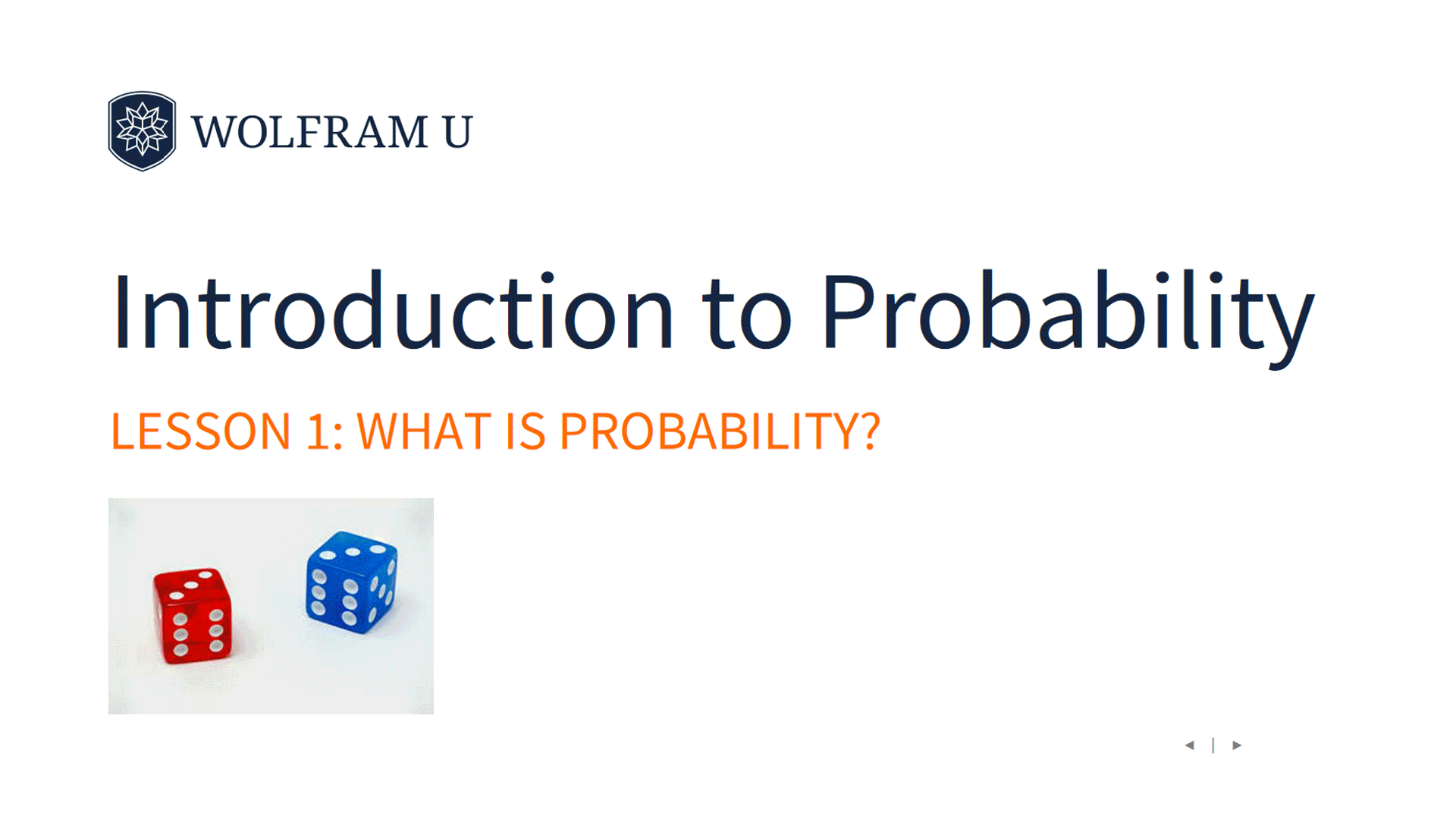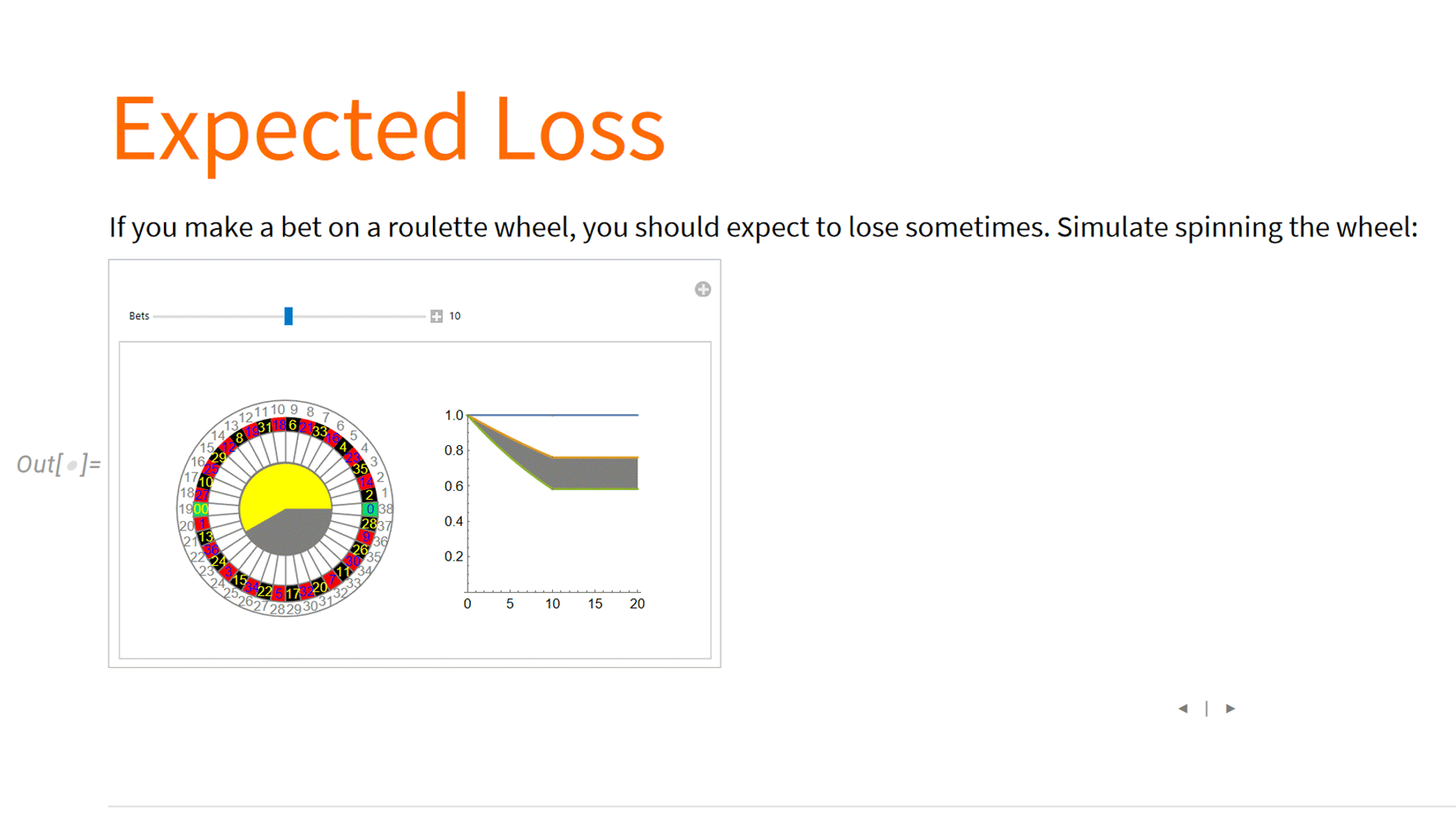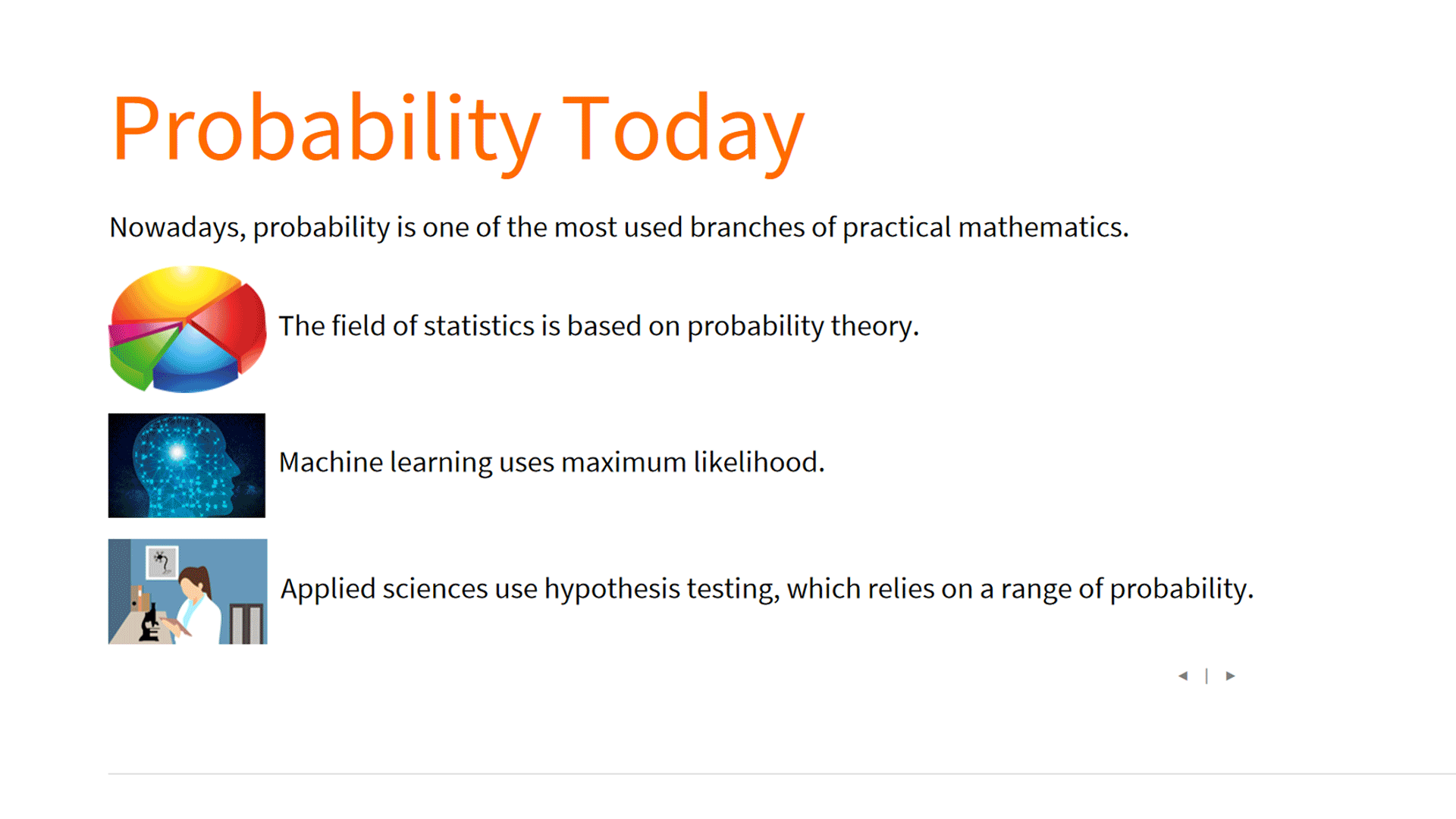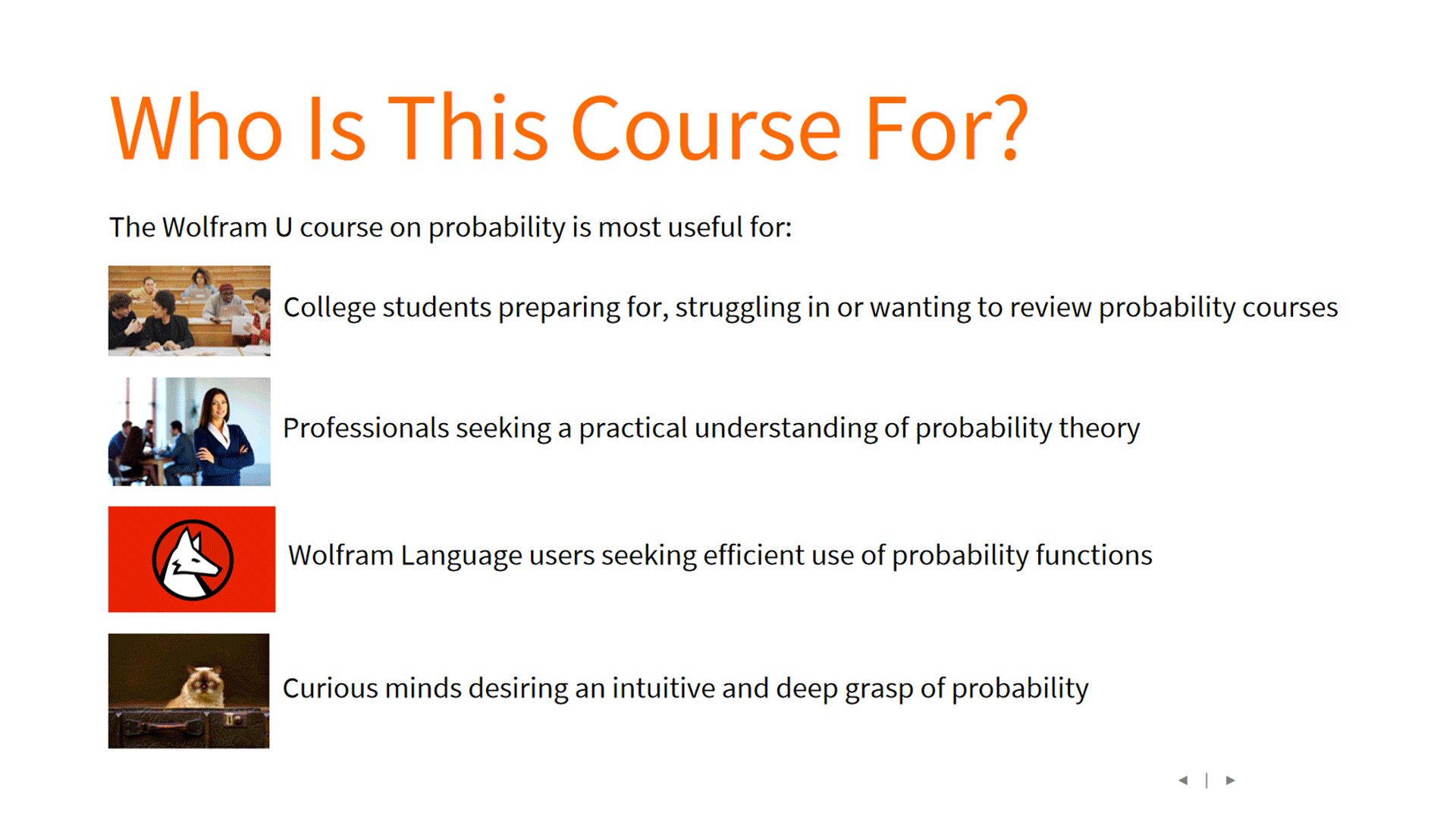
The full lesson notebook used in the video is also included so that you can try the code and interactive demonstrations for yourself. Any code in these notebooks can be copied with a simple click, and that code can be pasted into (and edited within) the scratch notebook area at the bottom of the screen.

Videos for each lesson are around eight minutes in length, but may vary depending on the requirements of the material—the video on joint distributions, for example, is the longest at 11 minutes, but it explores the most advanced and beautiful concepts of the course.

Exercises

Additionally, each lesson has a separate set of five exercises of increasing difficulty. All exercises also have solutions included. Exercises 1 and 2 test your direct understanding of the video; exercises 3 and 4 test your mathematical intuition on large problems; and exercise 5 pushes further than the lesson material, testing your deep grasp of the lesson. Doing the first three exercises is all that is needed to prepare for the quiz and final, but at least understanding the solution of exercise 5 may greatly help your comprehension.

Quizzes

Each of this course’s six sections ends with a 10-question multiple-choice quiz. Quiz questions are of comparable difficulty to exercises 2 or 3 of the lessons of that section, and anybody who does exercises 1 to 3 and reviews their solutions will probably (☺) pass the quiz without difficulty.

Students receive instant feedback upon submitting their responses to the quiz questions, and can use any reasonable method to arrive at the correct answer.

Course Certificate

Students who wish to take advantage of everything this course has to offer will, by the time they complete it, have watched all 25 lessons and passed the six quizzes. At this point, students can—and should!—request a certificate of completion showing their proficiency in the field of probability. This certificate can easily be added to your resume or social media profile too!

This course also has an optional final exam that you can take after completing all of the material. This final exam has more questions and a slightly higher difficulty than the quizzes, and passing it will net you a more advanced Level I Certification.

Feedback from the Daily Study Group

Wolfram U offered a glimpse of the course lessons and quizzes to Daily Study Group participants earlier this March, and we received some valuable feedback. Here is what participants said:

• “I appreciate the examples and applicability to real-life problems.”
• “The materials that are provided are very useful and informative. The framework that was set up for this course is also amazing.”
• “I really enjoyed this course. Thank you very much for putting so much effort into it and also for the nice course materials.”
• “Great information on the implications of probability theory on the 2008 financial crisis!”

A Building Block for Discovery

Randomness characterizes anything that we do not yet fully understand. Probability is there to predict the unpredictable, to navigate the storming sea of reality, to face the unknown. The strength of probability is the insights it provides once applied. If studied intently, this Introduction to Probability course will provide you with the knowledge and intuition necessary for success in whatever field you choose to pursue. Probably. ☺

Acknowledgments

This course is the result of the work of the Wolfram U team and the Algorithms R&D team. I would like to thank Devendra Kapadia, Anisha Basil, Joyce Tracewell, Abrita Chakravarty, Matt Coleman, Mariah Laugesen and Laura Crawford for all the work they put into getting this course up and running.